Math in… Fountains
You might have heard — or even derived in a physics class — that the water jets of a fountain and other projectiles create parabolic arcs.
A parabola is a U-shaped curve where every point is the same distance from a point (called a focus) and a straight line (called a directrix).
The usual derivation assumes the earth is flat and gravity pulls straight down.
However, the earth is round and gravity pulls towards its center, so the direction of acceleration of a projectile will change as it moves!
How does a round earth change the arc? First, let’s take a detour...
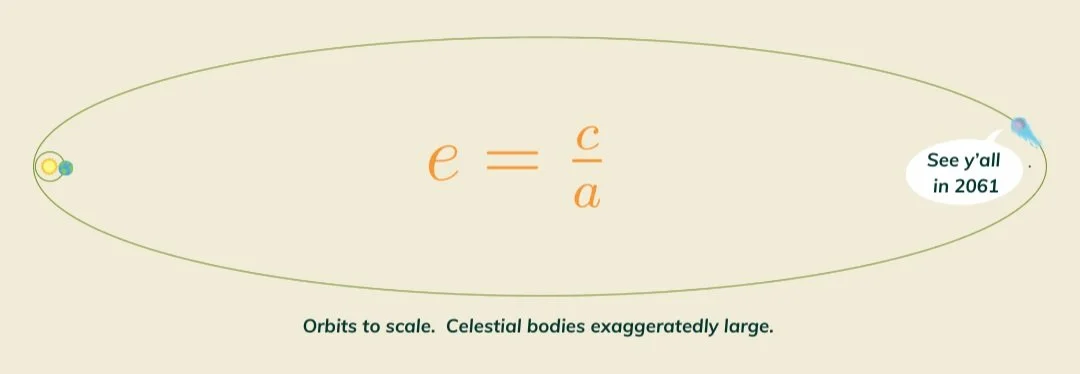
The closed gravitational orbit of a small object around a comparatively massive object, like a satellite around the earth or the earth around the sun, is approximately an ellipse if we ignore other sources of gravity.
Much like a circle is the set of points that all have the same radial distance from one point, called its center, an ellipse is the set of points that all have a common average distance from two points, called its foci.
In this model, an orbited body lies on one focus of the other body’s elliptical orbit. The earth’s elliptical orbit around the sun is almost circular, while Halley’s comet’s elliptical orbit around the sun has a higher eccentricity.
Much like the moon, the water drops of a fountain jet want to enter an elliptical orbit about the earth’s center of mass — but the pesky earth gets in the way!
As long as the projectile’s trajectory covers a short distance, a parabolic arc is a pretty great approximation for its elliptic arc, since we don’t really get to see the parts where the trajectories differ.
This elliptic arc is still an approximation for a projectile’s arc, though, since there are other forces acting on projectiles besides the earth’s gravitational pull.
And while it’s less important here than in celestial mechanics, in more rigorous models, orbits are technically about the barycenter of the two bodies and not just the greater center of mass. All we’re saying is that next time someone says a projectile’s arc is a parabola, don’t “um, actually” about an ellipse too hard!